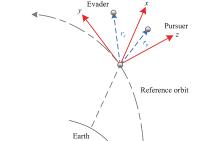
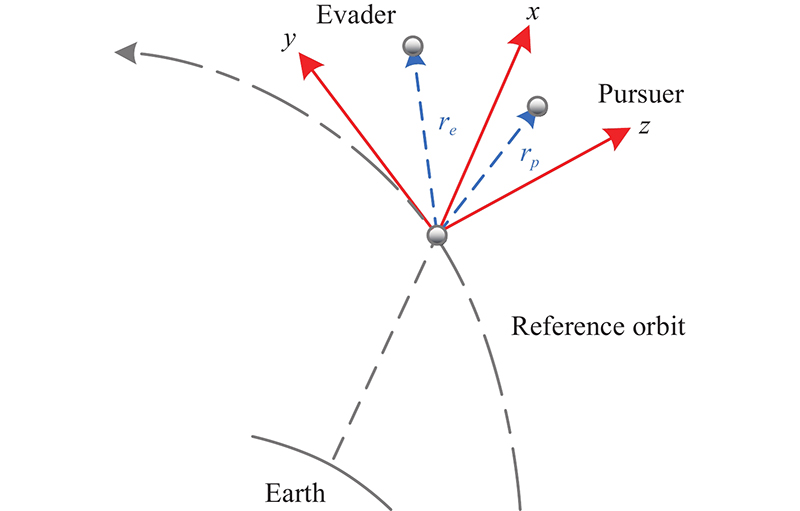
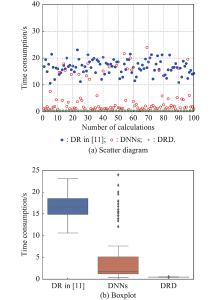
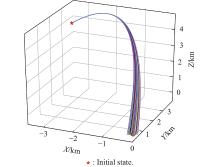
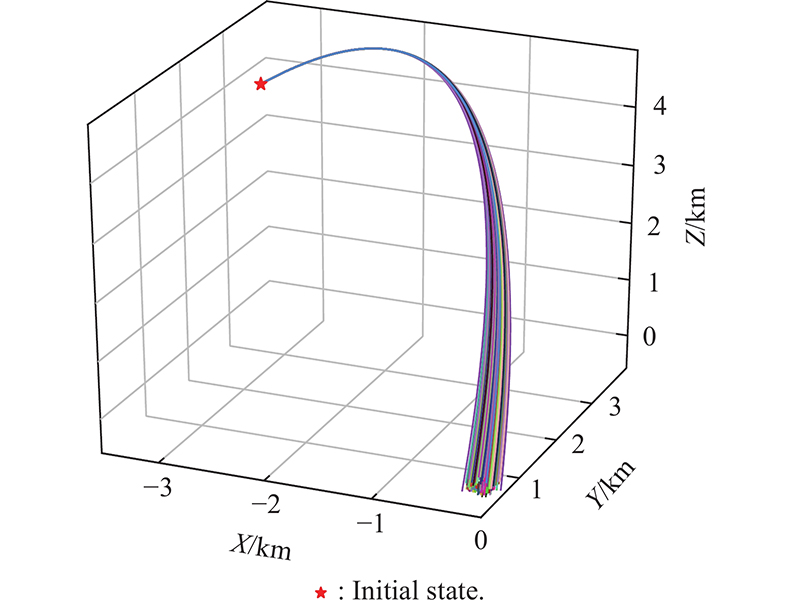
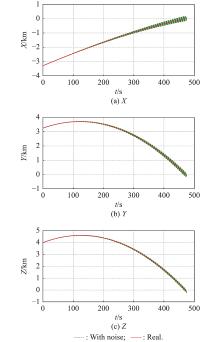
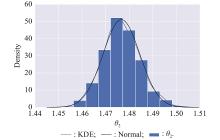
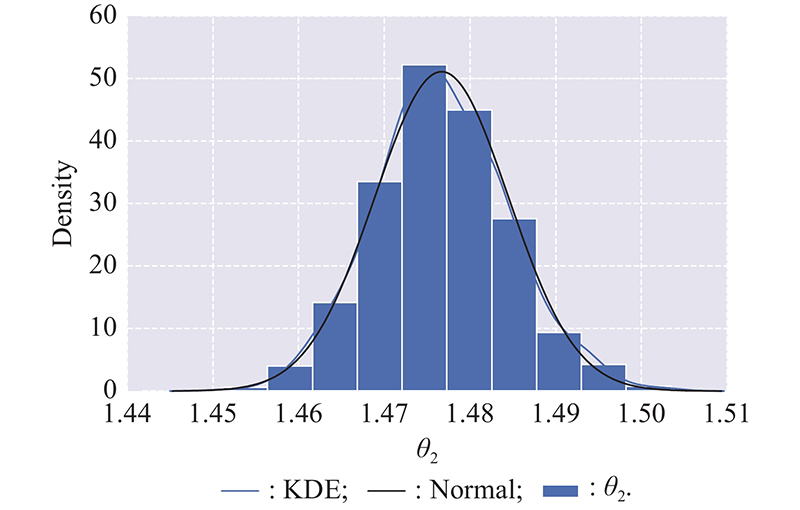
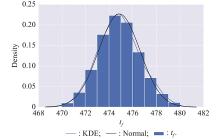
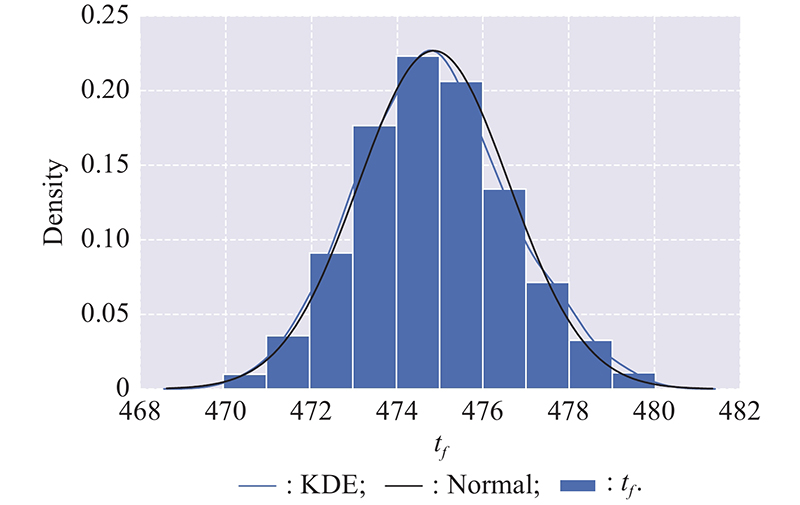
An optimal guidance method for free-time orbital pursuit-evasion game

doi:10.23919/JSEE.2022.000149

Journal of Systems Engineering and Electronics ›› 2022, Vol. 33 ›› Issue (6): 1294-1308.doi: 10.23919/JSEE.2022.000149

• • 上一篇

收稿日期:2021-04-25 出版日期:2022-12-18 发布日期:2022-12-24

An optimal guidance method for free-time orbital pursuit-evasion game

Chengming ZHANG(), Yanwei ZHU(), Leping YANG(), Xin ZENG()

¹ College of Aerospace Science and Engineering, National University of Defense Technology, Changsha 410000, China

Received:2021-04-25 Online:2022-12-18 Published:2022-12-24
Contact: Yanwei ZHU E-mail:zhchm_vincent@163.com;zywnudt@163.com;ylpnudt@163.com;xzavier0214@outlook.com
About author:
ZHANG Chengming was born in 1998. He received his B.S. and M.S. degrees from National University of Defense Technology (NUDT), Changsha, China, in 2019 and 2021, respectively. He is a graduate student with the College of Aerospace Science and Engineering, NUDT. His research interests include aerospace dynamics, guidance and control, and application of artificial intelligence to the control of astronautic systems. E-mail: zhchm_vincent@163.com

ZHU Yanwei was born in 1981. He received his B.S., M.S. and Ph.D. degrees from National University of Defense Technology (NUDT), Changsha, China, in 2002, 2004 and 2009, respectively. He is an associate professor with the College of Aerospace Science and Engineering, NUDT. His research interests include aerospace dynamics, guidance and control, and astronautic mission planning and design. E-mail: zywnudt@163.com

YANG Leping was born in 1964. He received his B.S. and M.S. degrees from National University of Defense Technology (NUDT), Changsha, China, in 1984 and 1987, respectively. He is a professor with the College of Aerospace Science and Engineering, NUDT. His research interests include aerospace dynamics, guidance and control, and astronautic mission planning and design. E-mail: ylpnudt@163.com

ZENG Xin was born in 1992. He received his B.S. and M.S. degrees from National University of Defense Technology (NUDT), Changsha, China, in 2014 and 2016, respectively. He is a student with the College of Aerospace Science and Engineering, NUDT. His research interests include aerospace dynamics, guidance and control, and application of artificial intelligence to the control of astronautic systems. E-mail: xzavier0214@outlook.com
Supported by:
This work was supported by the National Defense Science and Technology Innovation (18-163-15-LZ-001-004-13).

摘要/Abstract

Abstract:

With the development of space rendezvous and proximity operations (RPO) in recent years, the scenarios with non-cooperative spacecraft are attracting the attention of more and more researchers. A method based on the costate normalization technique and deep neural networks is presented to generate the optimal guidance law for free-time orbital pursuit-evasion game. Firstly, the 24-dimensional problem given by differential game theory is transformed into a three-parameter optimization problem through the dimension-reduction method which guarantees the uniqueness of solution for the specific scenario. Secondly, a close-loop interactive mechanism involving feedback is introduced to deep neural networks for generating precise initial solution. Thus the optimal guidance law is obtained efficiently and stably with the application of optimization algorithm initialed by the deep neural networks. Finally, the results of the comparison with another two methods and Monte Carlo simulation demonstrate the efficiency and robustness of the proposed optimal guidance method.

Key words: orbital pursuit-evasion, differential game, dimension-reduction, deep neural networks

. [J]. Journal of Systems Engineering and Electronics, 2022, 33(6): 1294-1308.

Chengming ZHANG, Yanwei ZHU, Leping YANG, Xin ZENG. An optimal guidance method for free-time orbital pursuit-evasion game[J]. Journal of Systems Engineering and Electronics, 2022, 33(6): 1294-1308.

图/表 29

参考文献 22

1	LUO Y Z, LI Z Y, ZHU H. Survey on spacecraft orbital pursuit-evasion differential games. Scientia Sinica Technologica, 2020, 50(12): 1533−1545.
2	ISAACS R. Differential games. New York: John Wiley & Sons, 1965.
3	RAIVIO T, EHTAMO H. Visual aircraft identification as a pursuit-evasion game. Journal of Guidance, Control, and Dynamics, 2000, 23(4): 701−708.
4	SHIMA T Optimal cooperative pursuit and evasion strategies against a homing missile. Journal of Guidance, Control, and Dynamics, 2011, 34 (2): 414- 425. doi: 10.2514/1.51765
5	PONTANI M, CONWAY B A Numerical solution of the threedimensional orbital pursuit-evasion game. Journal of Guidance, Control, and Dynamics, 2009, 32 (2): 474- 487. doi: 10.2514/1.37962
6	SUN S T. Two spacecraft pursuit-evasion strategies on low earth orbit and numerical solution. Harbin: Harbin Institute of Technology, 2015. (in Chinese)
7	YE D, SHI M M, SUN Z W. Satellite proximate pursuitevasion game with different thrust configurations. Aerospace Science and Technology, 2020, 99: 105715.
8	JAGAT A, SINCLAIR A J. Optimization of spacecraft pursuit-evasion game trajectories in the Euler-Hill reference frame. Proc. of the AIAA Guidance, Navigation, and Control Conference, 2014. DOI: 10.2514/6.2014-4131.
9	HAFER W T, REED H L, TURNER J D, et al. Sensitivity methods applied to orbital pursuit evasion. Journal of Guidance, Control, and Dynamics, 2015, 38(6): 1118−1126.
10	STUPIK J, PONTANI M, CONWAY B A. Optimal pursuit/evasion spacecraft trajectories in the hill reference frame. Proc. of the AIAA Guidance, Navigation, and Control Conference, 2012. DOI: 10.2514/6.2012-4882.
11	LI Z Y, ZHU H, LUO Y Z. A dimensionreduction solution of freetime differential games for spacecraft pursuitevasion. Acta Astronautica, 2019, 163: 201−210.
12	YIN S S, LI J, CHENG L Low-thrust spacecraft trajectory optimization via a DNN-based method. Advances in Space Research, 2020, 66 (7): 1635- 1646. doi: 10.1016/j.asr.2020.05.046
13	CHENG L, WANG Z B, JIANG F H, et al. Fast generation of optimal asteroid landing trajectories using deep neural networks. IEEE Trans. on Aerospace and Electronic Systems, 2020, 56(4): 2642−2655.
14	WU Q C, LI B, LI J, et al Solution of infinite time domain spacecraft pursuit strategy based on deep neural network. Aerospace Control, 2019, 37 (6): 13- 18.
15	LI Z Y, ZHU H, LUO Y Z Saddle point of orbital pursuit-evasion game under j2-perturbed dynamics. Journal of Guidance, Control, and Dynamics, 2020, 43 (9): 1733- 1739. doi: 10.2514/1.G004459
16	BASER T, OLSDER G J. Dynamic noncooperative game theory. Philadelphia: Society for Industrial and Applied Mathematics, 1999.
17	JIANG F H, BAOYIN H X, LI J F Practical techniques for lowthrust trajectory optimization with homotopic approach. Journal of Guidance, Control, and Dynamics, 2012, 35 (1): 245- 258. doi: 10.2514/1.52476
18	HOWARD D C. Orbital mechanics for engineering students. 2nd ed. Amsterdam: Elsevier, 2010.
19	CHENG L, WANG Z B, JIANG F H, et al. Real-time optimal control for spacecraft orbit transfer via multi-scale deep neural networks. IEEE Trans. on Aerospace and Electronic Systems, 2019, 55(5): 2436−2450.
20	CHENG L, JIANG F H, LI J F. A review on the applications of deep learning in aircraft dynamics and control. Mechanics in Engineering, 2020, 42(3): 267−276.
21	RUNARSSON T P, YAO X Search biases in constrained evolutionary optimization. IEEE Trans. on Systems, Man, and Cybernetics, Part C: Applications and Reviews, 2005, 35 (2): 233- 243.
22	VENIGALLA, SCHEERES D J. Spacecraft rendezvous and pursuit/evasion analysis using reachable sets. Proc. of the Space Flight Mechanics Meeting, 2018. DOI: 10.2514/6.2018-0219.

Bound	a /km	d /km
Lower bound	Re + 36500	1
Upper bound	Re + 36600	10

Coefficient	Value
DU/m	2 000
TU/s	400
VU/(m/s)	5

Layer	Unit
3	16, 32, 16
5	16, 32, 64, 32, 16
7	16, 32, 64, 128, 64, 32, 16
9	16, 32, 64, 128, 256, 128, 64, 32, 16

Result	DNNs	DRD
θ₁ /rad	−0.0253	−0.0248
θ₂ /rad	0.7867	0.7792
t_f /s	404.7246	404.7051
r_x /m	44.50	0.57
r_y /m	−41.20	−0.11
r_z /m	4.13	−0.09
t_c/ms	203.125	525.625

Result	DNNs	DRD
θ₁ /rad	0.0472	0.0470
θ₂ /rad	−0.6180	−0.6236
t_f /s	183.7244	183.8296
r_x /m	−4.1046	0.1955
r_y /m	−8.8362	0.1219
r_z /m	0.6038	−0.6262
t_c /ms	245.151	527.248

An optimal guidance method for free-time orbital pursuit-evasion game

RichHTML

PDF (PC)

可视化

摘要/Abstract

引用本文

使用本文

图/表 29

参考文献 22

相关文章 0

编辑推荐

Metrics

本文评价

Statistics	DRD	DNNs	DR in [11]
μ	0.4673	5.0134	16.6021
σ	0.0264	6.0634	2.6833
max	0.5469	23.9688	23.0562
min	0.3906	0.4188	10.6062

State	Real value	σ
r_x /m	−3292.165	32.922
r_y /m	3253.993	32.540
r_z /m	3990.389	39.904
v_x /(m/s)	8.897	0.089
v_y /(m/s)	7.506	0.075
v_z /(m/s)	9.964	0.100
n /s⁻¹	7.158×10⁻⁵	0.007×10⁻⁵

Statistics	Δr_x	Δr_y	Δr_z
μ	41.675	39.778	47.351
σ	31.388	28.834	35.812
max	187.835	151.409	237.766
min	0.112	0.017	0.020